
In the grand theater of mathematics, prime numbers and polynomials occupy center stage. These fundamental constructs have intrigued minds for centuries, leading to discoveries that underpin modern technology, from cryptography to advanced algorithms. This article examines recent research that delves into squarefree discriminants of polynomials with prime coefficients. Here, “squarefree” refers to numbers that are not divisible by any perfect square other than 1, such as 6 or 30 but not 36.
The study is not merely theoretical. Squarefree numbers appear in cryptographic protocols securing our bank transactions and personal messages. Polynomials with prime coefficients also find applications in data compression and numerical analysis. By exploring their discriminants — a polynomial’s key to distinguishing between distinct solutions — this research paves the way for deeper insights into algebraic structures and computational applications. Understanding squarefree discriminants opens pathways to unravel complex relationships between number theory and applied mathematics, bridging seemingly abstract principles with tangible, real-world benefits.
Key Insights into the Research
A Framework for Discriminants
Discriminants measure how “distinct” the roots of a polynomial are. A squarefree discriminant ensures that the polynomial’s roots do not overlap with multiplicity. For instance, the discriminant of is squarefree because its roots, 2 and -2, are distinct.
In this study, the authors extend this classical concept by considering families of polynomials with prime coefficients. They determine the density of such polynomials whose discriminants are squarefree as the polynomial degree increases. This density provides a measure of how common squarefree discriminants are within these polynomial families. The research employs advanced mathematical techniques, such as asymptotic density analysis and sieve theory, to uncover patterns that were previously inaccessible.
Prime Coefficients: A Special Case
The restriction to prime coefficients introduces new challenges and opportunities. While traditional integer-based polynomials have been widely studied, those with prime coefficients exhibit unique arithmetic properties. The research identifies patterns in these polynomials and connects them to local and global densities, crucial for understanding how often squarefree conditions are satisfied. The authors’ innovative approach bridges classical number theory with modern computational techniques, offering insights into how prime-based structures can model more complex systems.
Applications and Broader Impacts
- Cryptography: Polynomials with prime coefficients are foundational in creating secure keys for encryption algorithms. The uniqueness of primes ensures robust security mechanisms, making them invaluable in protecting sensitive data.
- Numerical Stability: Squarefree discriminants ensure that solutions to polynomial equations remain robust against numerical errors. Such stability is critical in simulations and models used in fields like fluid dynamics and climate science.
- Theoretical Physics: These structures often mirror phenomena in quantum mechanics and field theory, where prime-based formulations model symmetry and complexity. The mathematical framework proposed in this research could potentially influence the understanding of fundamental physical laws.
Below is a graph illustrating the asymptotic density of squarefree discriminants for polynomials of varying degrees (2 to 10). The density, as a percentage, decreases with increasing degree, reflecting the intricate conditions required for squarefree discriminants.
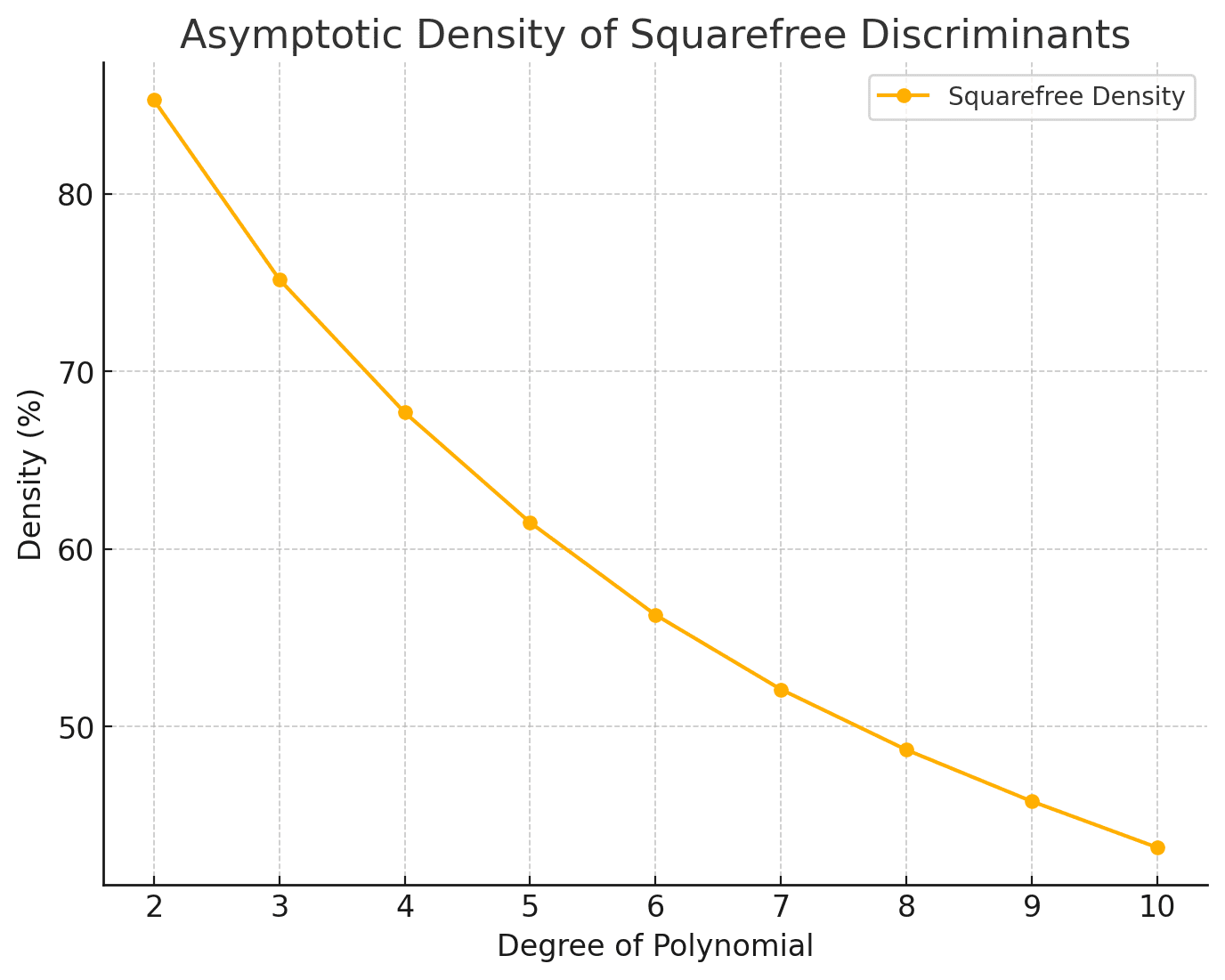
The graph underscores a fascinating trend: as the complexity of the polynomial increases, the constraints for achieving squarefree discriminants grow stricter. This reflects a broader theme in mathematics, where increased structure often leads to reduced generality.
The Prime Connection
Prime coefficients are more than a mathematical choice; they introduce a layer of uniqueness that mirrors cryptographic keys’ indivisibility. Each prime adds a distinct “flavor” to the polynomial, shaping its overall behavior and discriminant.
Squarefree Numbers in Action
Squarefree numbers are central to algorithms ensuring secure data transmissions, forming the backbone of blockchain and digital certificates. Their role extends beyond mathematics, influencing modern data architectures and protocols.
Impact on Algebraic Structures
The squarefree property stabilizes solutions, making it indispensable for designing efficient numerical solvers in engineering and physics. This stability ensures reliable results even in systems where precision is critical.
A Universal Limit
The density of squarefree discriminants approaches a specific limit (e.g., ~67.69% for certain families), offering a universal insight into polynomial behavior. This convergence highlights an underlying mathematical order amid apparent randomness.
Local Densities and Global Trends
This research reveals that the interplay between local densities (specific to primes) and global trends shapes the overall distribution of squarefree polynomials. Understanding these patterns could lead to advancements in fields as diverse as computational algebra and theoretical informatics.
Bridging Theory and Application
Exploring the Future of Squarefree Discriminants
Mathematics thrives in the interplay between the abstract and the tangible. The insights from this study are poised to influence areas as diverse as cryptography, data integrity, and theoretical physics. By anchoring future innovations in these findings, the mathematical community can further unravel the mysteries of prime-based structures.
The implications extend beyond academia. Engineers and scientists can leverage these insights to design systems that are not only robust but also efficient. From secure communication protocols to accurate computational models, the potential applications are vast and inspiring.
This ongoing dialogue between theory and application ensures that such research remains a beacon for progress, bridging the enigmatic beauty of primes with the practicalities of a digital world. As researchers continue to explore this fertile ground, new connections between prime-based mathematics and real-world problems will undoubtedly emerge, leading to breakthroughs that could redefine our understanding of both numbers and nature.
About Disruptive Concepts
Welcome to @Disruptive Concepts — your crystal ball into the future of technology. 🚀 Subscribe for new insight videos every Saturday!
See us on https://twitter.com/DisruptConcept
Read us on https://medium.com/@disruptiveconcepts
Enjoy us at https://disruptive-concepts.com
Whitepapers for you at: https://disruptiveconcepts.gumroad.com/l/emjml
New Apps: https://2025disruptive.netlify.app/







