Mathematics is rewriting its own rules — are you ready?
Numbers are the foundation of everything — from the structure of the universe to the algorithms that power our technology. But what if numbers aren’t as predictable as we once thought? What if hidden patterns in their behavior challenge our fundamental understanding of mathematics? This is precisely the case in number theory, where mathematicians seek to classify number fields, extensions of the rational numbers that define the very fabric of algebraic equations.
Counting number fields isn’t just an esoteric pursuit — it has profound implications. The way number fields grow underpins modern cryptography, the distribution of prime numbers, and the classification of algebraic structures. At the heart of this effort is Malle’s Conjecture, a bold prediction about how number fields should behave. But recent breakthroughs using inductive methods have revealed something startling: while Malle’s framework holds in many cases, it also fails in others. This means that our understanding of number fields — and by extension, the mathematical universe — is still incomplete.
The Mathematics of Hidden Structures
Number fields are extensions of the rational numbers that allow for deeper exploration into the fabric of algebra and arithmetic. In the grand game of counting these extensions, researchers have long relied on Malle’s Conjecture, which predicts the asymptotic number of field extensions with a given Galois group and bounded discriminant. However, despite its elegant formulation, Malle’s Conjecture has been met with puzzling inconsistencies.
Traditionally, methods for counting number fields required understanding Galois groups — the mathematical entities that describe how different field elements permute among themselves. What makes the new approach groundbreaking is its ability to work inductively, allowing mathematicians to build upon known results for simpler groups and apply them to more complex structures.
Instead of treating every number field in isolation, the researchers leverage the power of smaller, well-understood groups to make deductions about larger ones. This is akin to solving a jigsaw puzzle by starting with the edge pieces — once you understand the boundaries, you can infer the missing parts. This method has already led to numerous new cases of Malle’s Conjecture being proven, as well as unexpected counterexamples that expose the conjecture’s shortcomings.
Uncovering the Weaknesses in Malle’s Conjecture
While the new inductive approach validates many aspects of Malle’s Conjecture, it also provides counterexamples that prove certain cases were wrongly predicted. This reveals a striking truth: not all number fields behave as expected.
One of the key ideas is that permutation groups, which describe the symmetrical structure of number field extensions, do not always conform neatly to Malle’s predictions. The researchers found that some groups exhibit unexpected growth patterns when counting their associated number fields. In particular, they discovered that certain wreath products (complex combinations of smaller groups) lead to counterexamples where Malle’s predicted exponent for counting fields does not match reality.
A striking case is the C₃ ≀ C₂ group, which contradicts Malle’s conjectured growth rate. The researchers demonstrated that the number of such fields actually grows at a rate inconsistent with the conjecture’s strong form. This calls into question whether Malle’s original framework needs revision, especially when dealing with non-concentrated groups — those where the minimum index elements are not evenly distributed.
Inductive Reasoning and the Future of Number Field Counting
At the core of this discovery is the ability to systematically extend results from simpler cases to more complicated ones. The inductive method developed by the researchers relies on breaking down a complex number field into a tower of extensions, where each level of the tower contributes to the overall count in a predictable way.
A crucial aspect of their work involves abelian normal subgroups, which provide an entry point for applying these methods. By treating certain permutation groups as building blocks, they were able to extend known results and generalize counting techniques across a much wider range of groups.
To illustrate this visually, let’s consider the growth rate of number fields under this new approach. Below is a graph that shows the divergence between Malle’s predicted growth and the newly discovered cases:
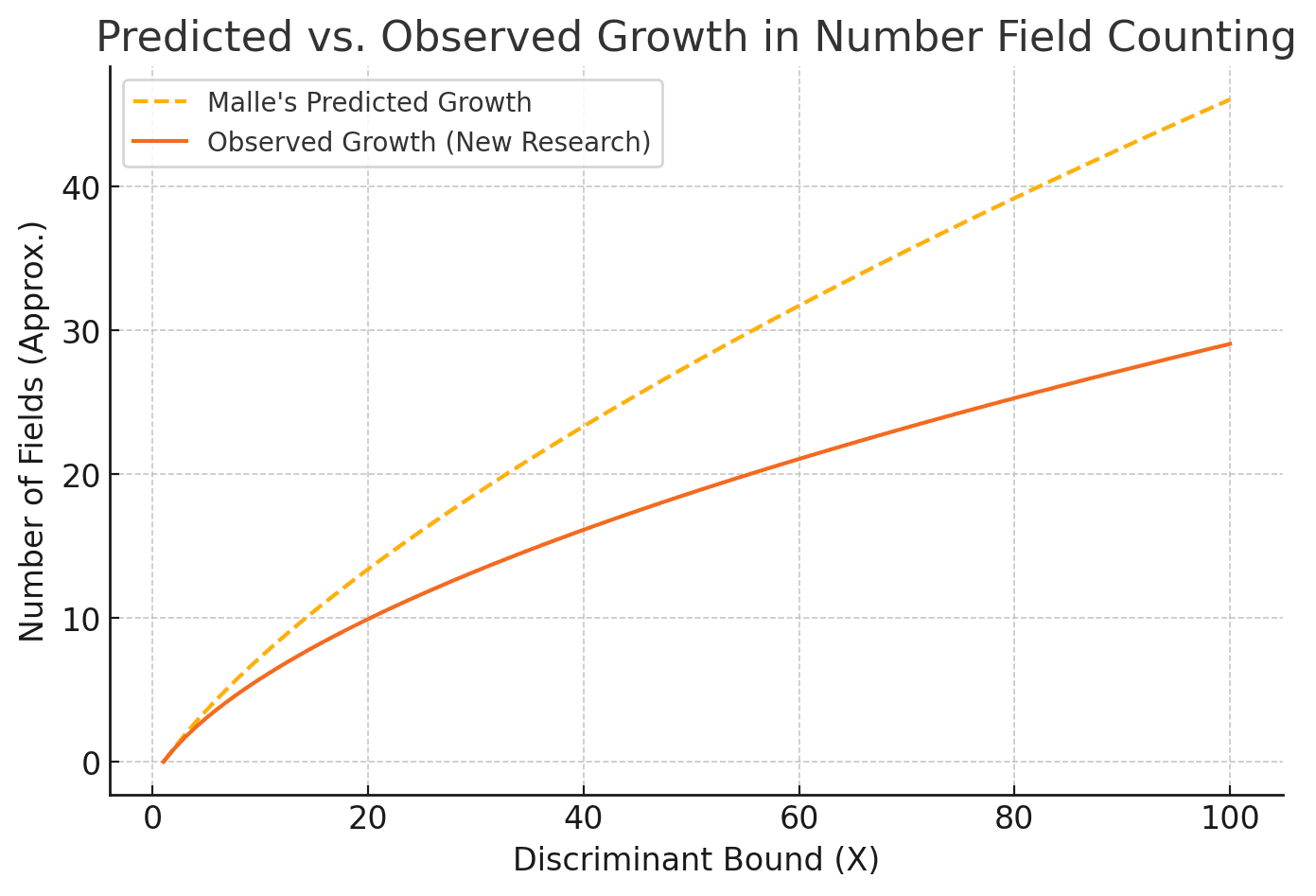
The graph above highlights how the new inductive method deviates from Malle’s original predictions, particularly in cases involving non-concentrated groups. This finding suggests that the conjecture may need to be reformulated for certain families of groups.
The Deeper Implications for Number Theory
Beyond the immediate impact on Malle’s Conjecture, these findings open doors to a broader mathematical revolution. If number fields can be counted more effectively through inductive methods, this could influence other areas of algebra and geometry, including:
- Class group analysis: Understanding the structure of ideal class groups in number fields.
- Arithmetic statistics: Predicting the distribution of prime numbers in algebraic settings.
- Cryptography: Refining security protocols that rely on the difficulty of certain number-theoretic problems.
In short, the new inductive framework is not just a technical improvement — it represents a paradigm shift in how we approach fundamental counting problems in mathematics.
The Counterexample That Changed Everything
Malle’s Conjecture predicted one growth rate for the C₃ ≀ C₂ group, but the new method proved it wrong, showing a totally different rate.
From Simple to Complex: The Inductive Leap
Using results from smaller groups, the researchers can now predict the behavior of far more complex field extensions.
The Power of Hidden Patterns
Number fields grow in seemingly random ways, but these researchers uncovered hidden symmetries that govern their behavior.
A Breakthrough in Counting Theory
This work extends known cases by an order of magnitude, allowing mathematicians to count fields they never could before.
The Unexpected Role of Wreath Products
Previously thought to follow Malle’s Conjecture, certain wreath products now appear to require a new mathematical framework.
Rewriting the Rules of Number Fields
Mathematics thrives on its ability to uncover hidden structures, to make sense of the infinite, and to reveal patterns that shape our understanding of reality. The new inductive method for counting number fields does more than just refine an old conjecture — it challenges us to think differently about the very nature of mathematical prediction. By proving new cases of Malle’s Conjecture and simultaneously uncovering counterexamples, this breakthrough forces us to ask deeper questions: How many other conjectures, assumed to be true, might need revision? What other hidden patterns are waiting to be discovered?
This is not just a technical advancement; it represents a shift in how we explore the universe of numbers. It reminds us that mathematics is still a living, evolving field, where the answers of yesterday may become the mysteries of tomorrow. The fact that we can now count number fields in ways that were once thought impossible speaks to the relentless ingenuity of human thought.
And this is just the beginning. What we’ve learned from these number fields might soon influence cryptography, quantum computing, and even the fundamental laws of physics. Each new discovery peels back another layer of the unknown, revealing a deeper, richer world beneath the surface.
If mathematics has taught us anything, it’s that the journey never truly ends — there is always another mystery to solve, another horizon to reach, and another equation waiting to change everything we thought we knew.
One thing is certain: the way we count number fields will never be the same again.
About Disruptive Concepts
Welcome to @Disruptive Concepts — your crystal ball into the future of technology. 🚀 Subscribe for new insight videos every Saturday!
See us on https://twitter.com/DisruptConcept
Read us on https://medium.com/@disruptiveconcepts
Enjoy us at https://disruptive-concepts.com
Whitepapers for you at: https://disruptiveconcepts.gumroad.com/l/emjml
New Apps: https://2025disruptive.netlify.app/







