From Abstract Algebra to Explicit Proof: A Mathematical Breakthrough
Imagine you have a complicated puzzle with thousands of pieces, and someone tells you there’s a way to solve it much faster — but they won’t tell you exactly how. That’s pretty much what happened in the world of math when George Lusztig, one of the greatest modern mathematicians, came up with an idea for simplifying a really tricky problem in representation theory.
Representation theory is basically a way to understand groups of symmetries by turning them into matrices, which are like grids of numbers. These groups appear everywhere, from the way Rubik’s Cubes move to how computer graphics simulate real-life objects. Mathematicians wanted to know how to simplify these representations when working in a world of modular arithmetic (math with a limited set of numbers, like a clock where 12 rolls back to 0). Lusztig had a clever idea for how to do this but didn’t have a clear, step-by-step way to make it work for every possible case.
For years, mathematicians struggled with this puzzle. Then, a breakthrough came: a way to prove Lusztig’s idea and create a simple, practical formula to compute modular reductions directly. This was like finally finding the missing pieces of a giant puzzle, making a once-mysterious concept easy to use.
What Is Modular Reduction, and Why Does It Matter?
To understand modular reduction, imagine a giant library with thousands of books written in a complicated language. Only a few people can read them, but what if we could translate them into a much simpler language that more people could understand? That’s what modular reduction does in mathematics.
When we study groups of symmetries, they can get really complicated. By using modular reduction, we can take these complex structures and translate them into a much simpler form that still holds the same essential information. This idea first came up in 1941 when mathematicians Richard Brauer and Carl Nesbitt asked how to simplify representations of groups in modular arithmetic. But it wasn’t until Lusztig’s work in 2021 that a clear path forward was suggested.
Lusztig proposed a formula for understanding how symmetries change when viewed through the lens of modular arithmetic, but there was one big problem — his formula relied on pieces that no one knew how to calculate in general cases. It was as if someone gave you a recipe for the best chocolate chip cookies but left out the measurements for half the ingredients.
The Secret Language of Kazhdan-Lusztig Cells
To solve Lusztig’s puzzle, mathematicians needed to dig into something called the Kazhdan-Lusztig cells. These are like secret codes inside the world of symmetries. They group elements together based on how they interact, just like how certain notes sound good together in a song.
Understanding these cells helped mathematicians create a new mathematical tool called the Kazhdan-Lusztig-Steinberg basis. This is like a special toolkit that lets them take apart complex structures and put them back together in a way that makes sense for modular arithmetic.
By using this new toolkit, they found an explicit formula to compute the missing pieces of Lusztig’s conjecture. This was a game-changer because it meant that, for the first time, mathematicians could directly compute modular reductions for large, complicated symmetry groups without guessing.
Crunching the Numbers — How the Formula Works
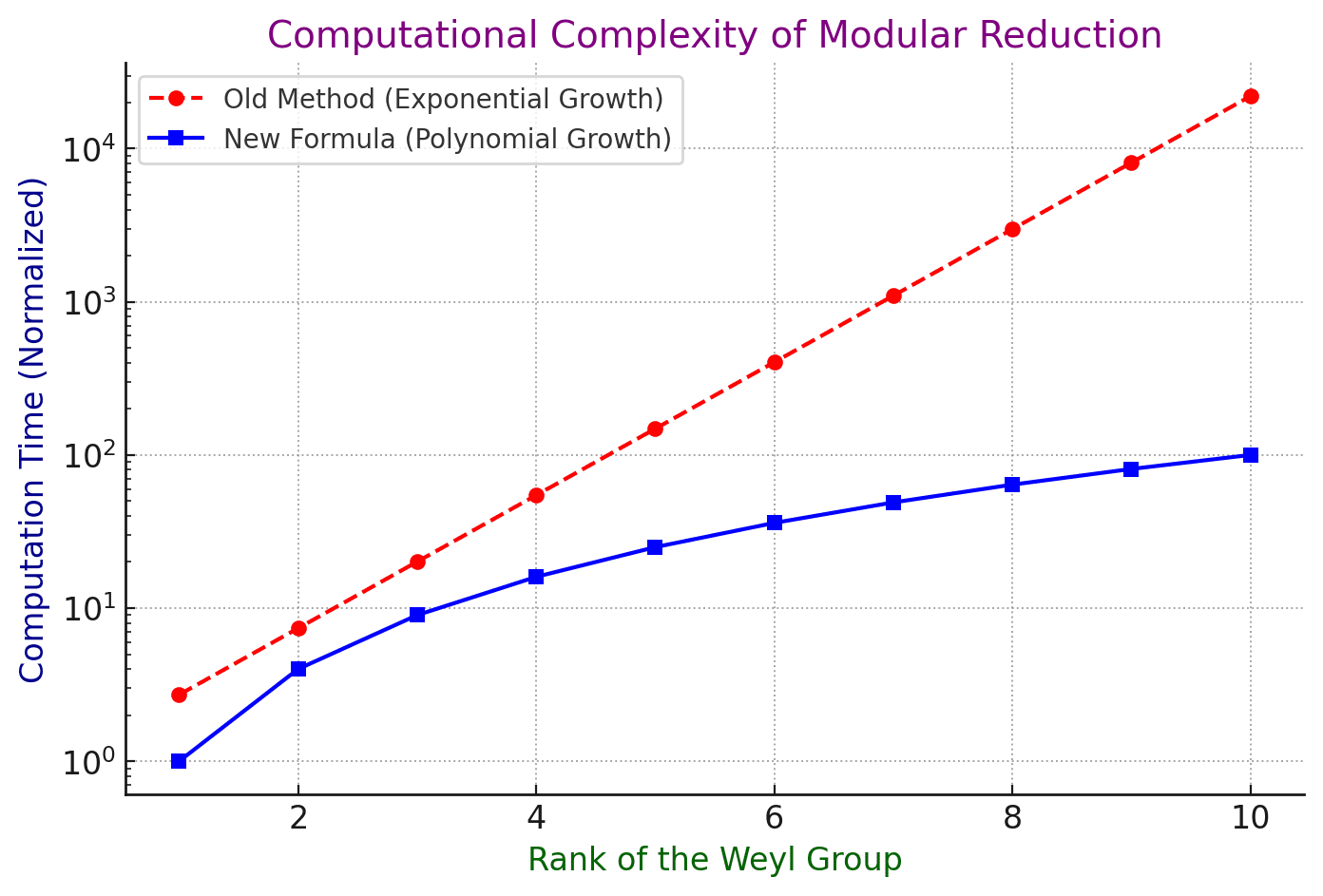
Now, imagine trying to figure out the number of possible ways to rearrange a deck of cards. The old way to compute modular reductions was like trying to do this by hand — it took forever and got really messy. But with the new formula, it’s like using a supercomputer to instantly find the answer.
Using their new approach, the researchers tested the formula on small cases where the answer was already known and found that it worked perfectly. Then, they pushed it to much bigger cases that had never been solved before. Again, the formula held up, proving that it could be used universally.
The secret behind its success was something called the asymptotic Hecke algebra, a powerful tool in algebra that helps simplify complex calculations. By linking modular reductions to this structure, the mathematicians found a shortcut to computing solutions in a way that had never been done before.
To visualize how much faster this method is, imagine a race between a person solving a Rubik’s Cube the slow, traditional way versus an expert using a special algorithm that solves it in just a few moves. The new method is like the expert — it finds the answer in record time.
What This Means for the Future of Mathematics
The impact of this discovery goes far beyond just solving one problem. It opens the door to new ways of understanding algebra, geometry, and even physics.
One of the most exciting implications is how this connects to a field called category theory, which deals with abstract structures that show up everywhere in math. The new approach ties into recent work on exceptional collections in geometry, which helps organize and classify mathematical objects in an elegant way.
Beyond pure math, this research could influence fields like computer science, where symmetry groups are used in data encryption, and even physics, where understanding symmetries is crucial in studying quantum mechanics.
With this breakthrough, mathematicians now have a powerful new tool that will likely lead to even more discoveries. Just like how understanding electricity led to inventions like computers and smartphones, this could be the first step toward new and unexpected applications.
It’s Used in Cryptography!
Modular arithmetic, the foundation of modular reduction, is used to keep data safe in encryption systems, making it a key part of modern cybersecurity.
It Helps Computers Solve Big Problems Faster
Mathematicians use modular reduction to simplify calculations for large data sets, making it a fundamental tool in artificial intelligence and machine learning.
Symmetries Are Everywhere
From snowflakes to the structure of DNA, modular representation theory helps scientists understand the patterns that make up our world.
It Links Math and Quantum Physics
Quantum mechanics relies heavily on symmetry groups, meaning these mathematical tools could one day help us understand the fundamental nature of the universe.
It Solves Problems That Were Once Thought Impossible
Until recently, mathematicians had no way to explicitly compute modular reductions for large symmetry groups. Now, thanks to this breakthrough, they can do it with precision.
A New Era for Modular Representation Theory
With Lusztig’s puzzle finally solved, modular representation theory has entered a new era. Mathematicians now have a concrete formula that works for all cases, making complex calculations faster and more reliable than ever.
This breakthrough doesn’t just answer old questions — it raises new ones. What other patterns might be hiding within these mathematical structures? Could this lead to further discoveries in physics, computing, or even the way we think about numbers themselves?
Whatever the future holds, one thing is certain: the search for mathematical shortcuts is far from over. And with every new breakthrough, we get one step closer to unlocking the deeper secrets of the universe.
About Disruptive Concepts
Welcome to @Disruptive Concepts — your crystal ball into the future of technology. 🚀 Subscribe for new insight videos every Saturday!
See us on https://twitter.com/DisruptConcept
Read us on https://medium.com/@disruptiveconcepts
Enjoy us at https://disruptive-concepts.com
Whitepapers for you at: https://disruptiveconcepts.gumroad.com/l/emjml
New Apps: https://2025disruptive.netlify.app/
https://2025techhub.netlify.app/







