When most of us think about mathematics, we imagine equations that have tidy answers and systems that behave predictably. But what happens when mathematicians discover something that doesn’t fit the mold? This is exactly what occurred when researchers delved into the world of GL-algebras — structures governed by the general linear group, a concept that is key to understanding symmetries in mathematical objects. For decades, these algebras were thought to follow the rules of Noetherianity, a principle that implies a certain neatness to their behavior. But the recent discovery of non-Noetherian GL-algebras in fields with characteristic two has turned this assumption on its head, revealing a type of algebra that grows endlessly, defying the tidy expectations of classical mathematics.
The Infinite Ascent
At the heart of this discovery is a simple yet powerful idea: under specific conditions, GL-algebras do not behave as expected. Traditionally, Noetherianity has served as a cornerstone in the study of algebraic structures, offering a sense of control and predictability. In this context, a Noetherian algebra is one where ascending chains of ideals eventually stabilize, meaning that after a certain point, no new or larger structures can form. However, recent research has shown that in characteristic two, an infinite ascending chain of GL-stable ideals can persist without stabilization. This revelation not only challenges long-held beliefs but also opens the door to a new understanding of algebraic structures in mathematics, highlighting the rich and surprising complexity of GL-algebras.
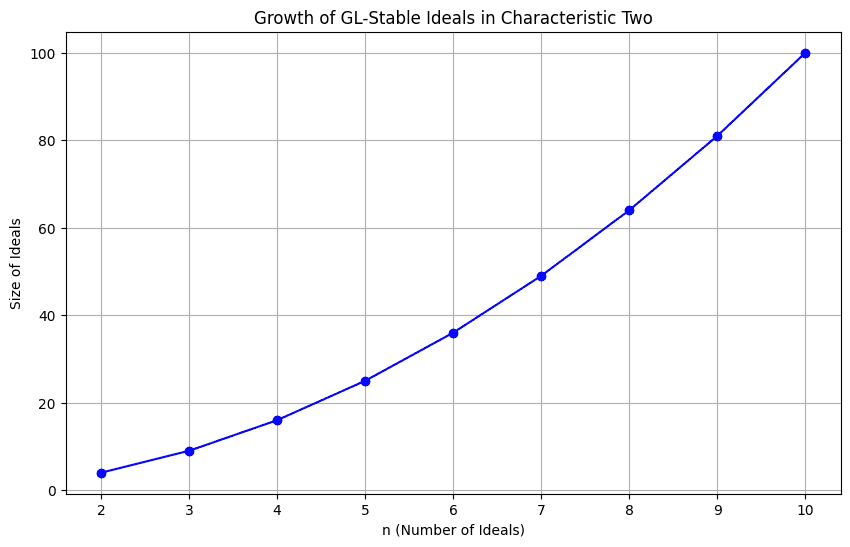
In the exploration of non-Noetherian GL-algebras, particularly those in characteristic two, one of the most striking features is the infinite growth of GL-stable ideals. Unlike in typical algebraic structures where these chains stabilize, in this case, the ideals continue to grow without limit. To better understand this phenomenon, the following graph visually depicts the progression of these ideals as they expand. It highlights how, as the number of ideals (n) increases, the size of each ideal also increases exponentially, reinforcing the concept of their non-stabilizing nature. This graph serves as a powerful visual tool to grasp the uniqueness and complexity of these algebraic structures.
The Implications of Non-Noetherian GL-Algebras
The discovery of non-Noetherian GL-algebras in characteristic two isn’t just a quirky mathematical oddity — it has profound implications for both theoretical mathematics and practical applications. For one, it challenges the very foundations of what mathematicians thought they knew about algebraic structures. This has potential ripple effects across various fields, including coding theory, cryptography, and even theoretical physics, where the behavior of algebraic systems underpins the development of new technologies. Additionally, the fact that these GL-algebras can continue to grow infinitely means that there are new, unexplored territories in the world of algebra waiting to be understood, each holding potential insights into the fabric of mathematics itself.
A New Frontier in Algebra
As mathematicians continue to explore the boundaries of GL-algebras, the discovery of their non-Noetherian counterparts marks the beginning of a new frontier in algebra. This uncharted territory is not just an academic curiosity; it represents a shift in how mathematicians approach the study of algebraic structures. The implications of this discovery are vast, suggesting that there are more “non-behaving” algebras out there, waiting to be discovered and understood. As researchers dive deeper into this field, they are likely to uncover more surprises, leading to new theorems, applications, and perhaps even a redefinition of some of the basic principles that have guided mathematics for centuries.
The Infinite Growth of GL-Algebras
Unlike most mathematical objects that reach a stopping point, non-Noetherian GL-algebras continue to grow infinitely without stabilizing. This defies the traditional understanding that every algebra should eventually settle into a stable structure. This discovery challenges and expands our understanding of mathematical infinity.
Characteristic Two’s Unique Role
The behavior of non-Noetherian GL-algebras is closely tied to the field characteristic, specifically characteristic two. In this setting, the usual rules of algebra break down, allowing these algebras to exhibit their infinite growth. This highlights the significance of field characteristics in determining the behavior of algebraic systems.
A Challenge to Classical Theorems
The discovery of non-Noetherian GL-algebras in characteristic two presents a direct challenge to longstanding theorems in algebra. These theorems, once thought to be universally applicable, now require reevaluation in light of this new evidence, showing that mathematical truths can be more flexible than previously believed.
Implications for Technology
Beyond pure mathematics, the behavior of these GL-algebras has potential implications for technology, particularly in fields like cryptography and coding theory. The ability of these algebras to grow infinitely could lead to new ways of encoding information or securing data, pushing the boundaries of current technological capabilities.
A New Path in Mathematical Research
The discovery has opened a new path for mathematical research, encouraging mathematicians to explore other areas where traditional assumptions might not hold. This could lead to further discoveries that change the way we understand the mathematical world, demonstrating that even well-established fields have new frontiers to explore.
Hopeful Horizons
The discovery of non-Noetherian GL-algebras in characteristic two is not just a puzzle for mathematicians to solve — it represents a glimpse into the endless potential of mathematical exploration. This breakthrough shows that even in the most established fields, there is always more to discover, more questions to ask, and more answers to uncover. As researchers continue to push the boundaries of what we know, the infinite growth of these algebras serves as a metaphor for the limitless possibilities that await those who dare to explore the unknown. With each new discovery, we come one step closer to understanding the profound and intricate beauty of the mathematical universe, and this journey is just beginning.
About Disruptive Concepts
https://disruptive-concepts.com/
Welcome to @Disruptive Concepts — your crystal ball into the future of technology. 🚀 Subscribe for new insight videos every Saturday!
Discover the Must-Have Kitchen Gadgets of 2024! From ZeroWater Filters to Glass Containers, Upgrade Your Home with Essential Tools for Safety and Sustainability. Click Here to Transform Your Kitchen Today!







