A new method simplifies complex quantum simulations.
Imagine if we could predict the behavior of molecules or design new materials using quantum computers. That’s the promise of quantum simulation, but there’s a catch: it’s really, really hard. Traditional methods, like Suzuki-Trotter formulas, need an overwhelming number of operations, especially when trying to achieve high precision. But there’s good news! A new method called Stochastic Zassenhaus Expansions (SZEs) changes the game. By cleverly breaking down the math and using smart shortcuts, SZEs make quantum simulations much more efficient. Let’s explore what makes them so exciting.
The Problem with Old Methods: Why We Need SZEs
Quantum simulations help us solve important problems, like figuring out how molecules interact or improving materials for technology. The issue? Traditional methods take way too many steps, especially as the systems get larger or more accurate answers are needed.
SZEs fix this problem. Instead of doing the same steps over and over, SZEs use a smarter approach. They break down the simulation into smaller parts using something called the Zassenhaus formula. This method not only saves time but also uses fewer resources, which makes it perfect for today’s quantum computers.
What Makes SZEs Different: A New Way to Simplify
At the heart of SZEs is the Zassenhaus formula. Think of it like a recipe that breaks a complex task into simpler steps. SZEs take this recipe and improve it by focusing on only the most important parts. For example, in a quantum system, SZEs use special operations called commutators to create high-accuracy results without adding extra work.
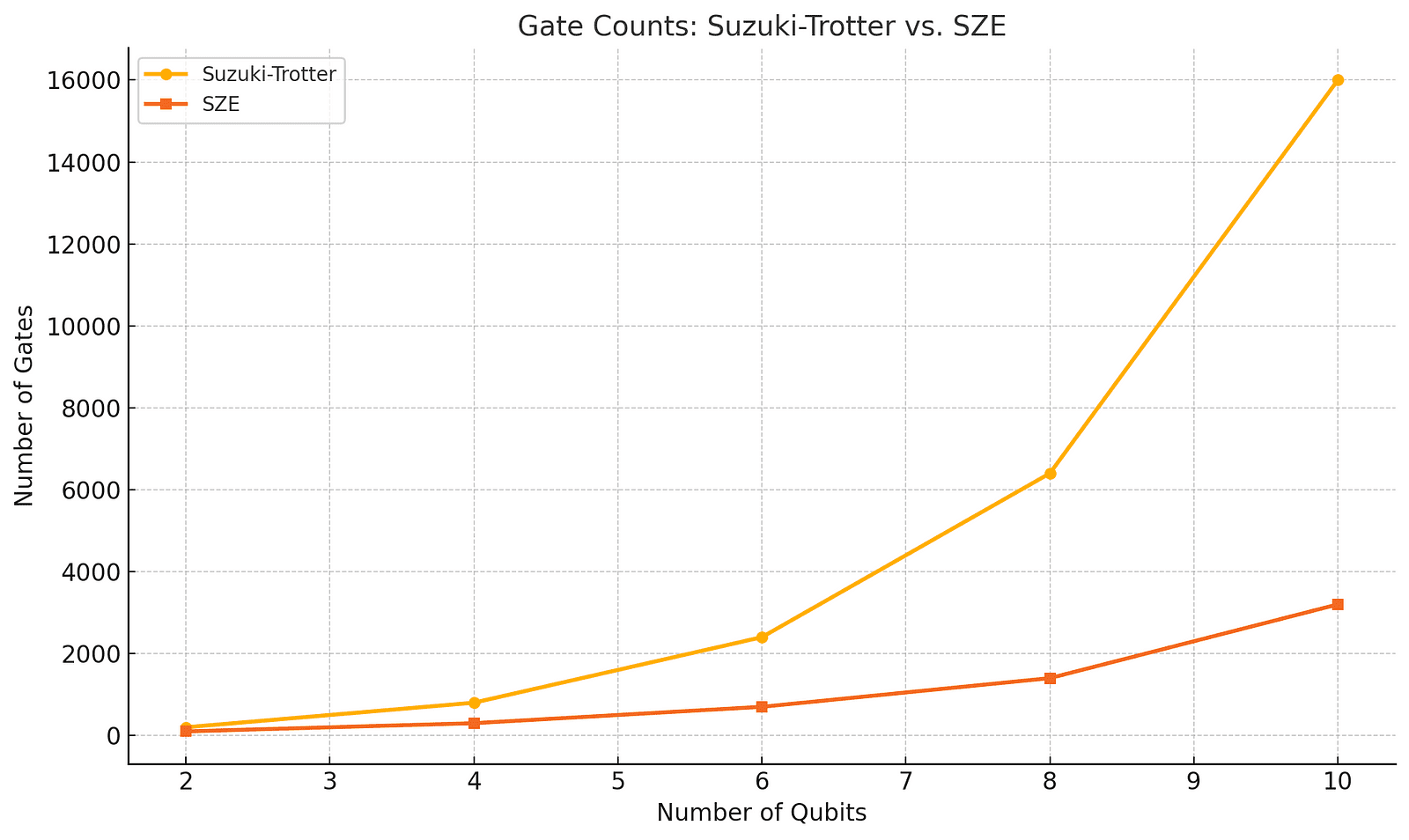
In practical terms, SZEs have already proven they work better. For a type of quantum simulation called the transverse-field Ising model, SZEs reduced the number of gates (steps in the process) by 42 times compared to traditional methods. This means they’re not just theoretical — they actually work in real life and save a ton of resources.
Using Probability to Save Time
One of the coolest things about SZEs is how they use randomness. Instead of calculating every possible outcome, SZEs sample or guess certain parts to save time. This is a bit like estimating how many jellybeans are in a jar instead of counting each one.
For example, in a simulation with 10 qubits (smallest units of quantum data), traditional methods repeat the same steps again and again. SZEs, however, only focus on the most critical parts and estimate the rest. This approach drastically reduces the amount of work needed and still provides highly accurate results. In fact, SZEs often produce fewer errors than older methods, making them both faster and better.
Below is a graph showing how SZEs use far fewer gates than traditional Suzuki-Trotter methods as systems grow larger.
They Focus on What Matters Most
SZEs use a clever formula to avoid unnecessary calculations, achieving high precision without wasting resources.
They Mimic How Nature Works
By using randomness, SZEs align perfectly with the natural probabilistic nature of quantum systems.
Proven Success in Real Examples
SZEs have been tested on important quantum problems and shown to drastically reduce errors compared to old methods.
Perfect for Today’s Quantum Computers
SZEs work well with the limitations of current quantum hardware, helping us take practical steps toward advanced computing.
A Simple Way to Solve Complex Problems
SZEs combine straightforward math with innovative techniques, making them easier to implement than many other methods.
A Bright Future for Quantum Simulations
Quantum computing is full of possibilities, but it’s also full of challenges. With tools like stochastic Zassenhaus expansions, those challenges are starting to fade. SZEs make it possible to simulate complex quantum systems more efficiently and with fewer errors. This not only opens the door for faster scientific discoveries but also makes quantum computing accessible for a wider range of applications. As we continue to innovate, methods like SZEs remind us how simple ideas can make a huge difference in solving some of the hardest problems in science and technology.
About Disruptive Concepts
Welcome to @Disruptive Concepts — your crystal ball into the future of technology. 🚀 Subscribe for new insight videos every Saturday!
See us on https://twitter.com/DisruptConcept
Read us on https://medium.com/@disruptiveconcepts
Enjoy us at https://disruptive-concepts.com
Whitepapers for you at: https://disruptiveconcepts.gumroad.com/l/emjml
New Apps: https://2025disruptive.netlify.app/







